Answer:
The correct option is B) all real numbers.
Explanation:
Consider the provided inequity.

We need to solve the inequity for n.


Subtract 3n from both sides
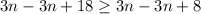

Which is true for any real number. As 18 is greater than 8.
Hence, the value of n is all real numbers.
Thus the correct option is B) all real numbers.