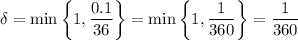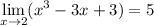
is to say that for any
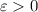
, we can find
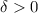
that guarantees
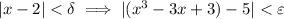

Observing that
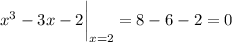
, the polynomial remainder theorem tells us that we can factorize the cubic to get
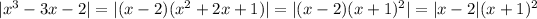
If we assume
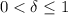
, we can set up a corresponding upper bound on the quadratic factor. We start with
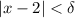
, from which we have

Now,
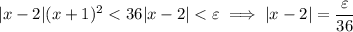
which suggests that we can choose
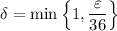
to ensure that we arrive at the inequality
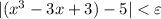
.
So, given
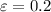
, we would have
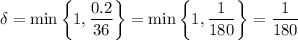
If
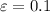
, we would take