Answer:
1. 1
3. 729/8
Step-by-step explanation:
Part 1
In the sequence: 729, -243, 81,...
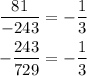
• The first term, a = 729
,
• The common ratio, r = -1/3
The nth term of a geometric progression is obtained by the formula below:
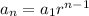
Therefore, the rule for the nth term will be:
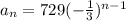
We then find a7, the seventh term.
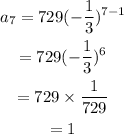
The seventh term is 1.
Part 3
In the sequence: 8, 12, 18,...
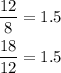
• The first term, a = 8
,
• The common ratio, r = 1.5
The nth term of a geometric progression is obtained by the formula below:
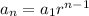
Therefore, the rule for the nth term will be:
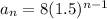
We then find a7, the seventh term.
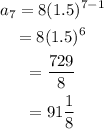
The seventh term is 729/8.