Question

Find the initial value P, growth/decay factor a, and growth/decay rate r for the following exponential function: Q(t) = 725 (1.07)^tNeed helping finding growth rate R = (a) The initial value is P= 725B) (b) The growth factor is a =1.07(c) The growth rate is r=(Note that if r gives a decay rate you should have r < 0.)

Answer 1

The growth rate of the function is 0.07

Solution

We are given a growth function Q(t) defined by:

`Q(t)=725(1.07)^t`

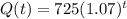
- We are required to find the growth rate of the function.

- The growth rate of the function and the growth factor of the function share a relationship given below:

`\begin{gathered} a=1+r \\ \text{where,} \\ a=\text{growth factor} \\ r=\text{growth rate} \end{gathered}`

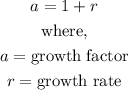
- We know that the growth factor is 1.07, thus, we can find the growth rate as follows:

`\begin{gathered} a=1+r \\ 1.07=1+r \\ \text{Subtract 1 from both sides} \\ 1.07-1=1-1+r \\ \\ \therefore r=0.07 \end{gathered}`

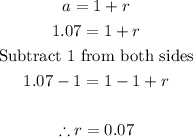
Final Answer

The growth rate of the function is 0.07