Question

Billy Gates wants ₱5,000 at the end of each 3-month period for the next 6 years. If Billy’s bank is paying 8% interest compounded quarterly, how much must she deposit if she wants the payments at the beginning of each 3-month period.

Answer 1

Given:

The final amount is given as A = ₱5,000.

The number of yeats is T = 6.

The interest is compounded at the end of each 3 months, n = 4 per year.

The rate of interest is r = 8% = 0.08.

The objective is to find the amount deposited at the beginning.

Step-by-step explanation:

The general formula to find the compound interest is,

`\begin{gathered} A=P(1+(r)/(n))^(nt)_{} \\ P=\frac{A}{(1+(r)/(n))^(nt)_{}}\text{ . . . . . . . (1)} \end{gathered}`

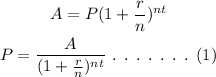
On plugging the given values in equation (1),

`\begin{gathered} P=(5000)/((1+(0.08)/(4))^(4(6))) \\ P=(5000)/((1+0.02)^(24)) \\ P=(5000)/((1.02)^(24)) \\ P=3108.607439\ldots\text{..} \\ P\approx3108.61 \end{gathered}`

Hence, the amount to be deposited is ₱3108.61.