Question

Birdie Brunella wants ₱5,000 at the end of each 3-month period for the next 6 years. If Birdie’s bank is paying 8% interest compounded quarterly, how much must she deposit now in order to receive the desired ordinary annuity?

Answer 1

Given:

Birdie Brunella wants $5,000 at the end of each 3-month period for the next 6 years. If Birdie’s bank is paying 8% interest compounded quarterly.

We will find the initial deposit should be made now

so,

A = $5,000 * 6 * 12/3 = 120,000

Compunded quarterly, n = 4

Time = 6 years

interest rate = 8% = 0.08

The compounded interest formula is:

`A=P\cdot(1+(r)/(n))^(nt)`
`\begin{gathered} 120000=P\cdot(1+(0.08)/(4))^(4\cdot6)=P\cdot1.6084 \\ \\ P=(120000)/(1.6084)=74,606.58 \end{gathered}`

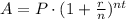
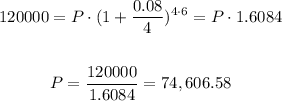
so, the answer will be $74,606.58