Question

A wheel rotating at 2000 rpm is braked and comes to rest in 30 seconds. How many revolutions did the wheel rotate through before coming to rest?

Answer 1

ω = 2000 rpm, initial angular speed.

`\omega = (2000 \, (rev)/(min) )*(2 \pi \, (rad)/(rev) )*( (1)/(60)\, (min)/(s) ) = 209.4395 \, (rad)/(s)`
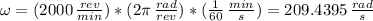
t = 30 s, the time for the wheel to come to rest.

Calculate the angular deceleration, α.
w - αt = 0
(209.4395 rad/s) - (α rad/s²)*(30 s) = 0
α = 6.9813 rad/s²

The angular distance traveled, θ, is given by
ω² - 2αθ = 0
θ = ω²/(2α)
= 209.4395²/(2*6.9813)
= 3141.6 rad

The number of revolutions is
3141.6/(2π) = 500

Answer: 500 revolutions