The given point is (-2,1) and the given line is x-3y=5.
The slope of the given line can be determined as,
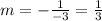
The equation of the line which is parallel to the given line will have the same slope.
Thus, the equation of the new line passing through (-2,1) can be determined as,
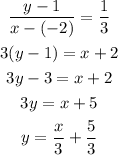
Thus, the equation of the line in slope intercept form is y=x/3+5/3.
The equation of the line in the point slope form can be determined as,
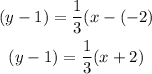
Thus, the equation of the line in the point slope form is (y-1)=(x+2)/3