Question

A 226.4-l cylinder contains 65.5% he(g) and 34.5% kr(g) by mass at 27.0°c and 1.40 atm total pressure. what is the mass of he in this container?

Answer 1

Answer : The mass of helium (He) in this container is, 0.2 grams

Solution :

First we have to calculate the moles of gas present in the container.

Using ideal gas equation,

`PV=nRT\\\\n=(RT)/(PV)`

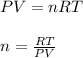
where,

P = pressure of the gas present in the container = 1.40 atm

V = volume of the gas present in the container = 226.4 L

R = gas constant = 0.0821 Latm/moleK

T = temperature of the gas =
`27^oC=273+27=300K`

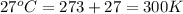
n = moles of gas present in the container = ?

Now put all the given values in the above equation, we get the moles of gas present in the container.

`n=((0.0821Latm/moleK)* (300K))/((226.4L)* (1.40atm))=0.077moles`

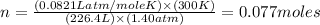
Now we have to calculate the moles of helium.

`\text{Moles of helium}=0.077* (65.5)/(100)=0.050mole`

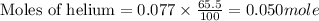
Now we have to calculate the mass of helium present in the container.

`\text{Mass of helium}=\text{Moles of helium}* \text{Molar mass of helium}=0.050moles* 4g/mole=0.2g`

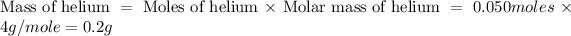
Therefore, the mass of helium (He) in this container is, 0.2 grams