Question

Determine if the expression -c? + 2c4 is a polynomial or not. If it is a polynomial,state the type and degree of the polynomial.

Answer 1

A polynomial is an algebraic expression of ordered addition, subtraction, and multiplication of variables, constants, and exponents. A polynomial can have more than one variable (x, y, z), constants (integers or fractions), and exponents (which can only be positive integers).

Then, let us see examples of what a polynomial is and is not:

• They are not polynomials

`(2)/(x+2)`

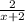
Because the variable cannot be in the denominator

`3x^(-2)`

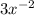
Because the exponent is -2 and the exponents can only be 0,1,2, ..., etc.

`\sqrt[]{x}=x^{(1)/(2)}`

Because the exponent cannot be a fraction

• They are polynomials

`\begin{gathered} 3x \\ x-2 \\ (3)/(8)x+x^2+5x^3+18x^6 \end{gathered}`

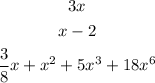
According to the above, the given expression is a polynomial because it is a sum of a variable with integer and positive exponents. Also, the variable is not in the denominator of any fraction.

• Now, according to the ,number of terms, in a polynomial, there are ,3 types of polynomials,. They are ,monomial, binomial and trinomial,. For example:

`\begin{gathered} 3x^2\Rightarrow\text{monomial} \\ 2x+7\Rightarrow\text{ binomial} \\ 5x+8y-4z+6w-3\Rightarrow\text{ polinomial} \end{gathered}`

In this case, the given expression has two terms, and then it is a binomial.

Finally, the degree of a polynomial is the highest exponent value of any of its terms.

Therefore, the degree of the given polynomial is 4.