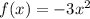Question

Quadratic Functions write the equation for each of the following. start with the graph of f(x) =x²1- reflect over the x-axis, stretch by a factor of 3

Answer 1

Step 1. We start by looking at the graph of the function:

`f(x)=x^2`

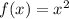
The graph is:

Step 2. The horizontal axis is the x-axis, and to reflect the original graph (the graph from step 1) over the x-axis, we add a negative sign next to the x^2:

`f(x)=-x^2`

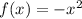
We can look at the resulting graph to confirm that the graph has now been reflected over the x-axis:

Step 3. We have reflected the graph over the x-axis but we still need to stretch it by a factor of 3.

We can stretch the graph of a quadratic function by multiplying the squared term by the factor, in this case by 3.

The resulting equation is:

`f(x)=-3x^2`

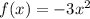
And the graph will finally look as follows:

Answer:

`f(x)=-3x^2`