Question

What is the equation of the line that would pass through the points graphed below?

Answer 1

the equation of the line can be written in slope-intercept form as

`y=mx+b_{}`

To get the equation of the line, solve for the value of m and b.

Based on the given plot, the first point (lower point) has the coordinates (-3,-3) while the second point (higher point) has the coordinates (3, 5). We need these to solve for the slope of the line. The slope of the line can be computed using the equation

`m=\frac{y_2-y_1_{}}{x_2-x_1}`

Substitute the values on the equation above based on the coordinates of the two points and compute

`m=\frac{5-(-3)_{}}{3-(-3)_{}}=(8)/(6)=(4)/(3)`

What's left to solve is the value of b. To solve this, use one of the coordinates and input the values on the slope-intercept form of the equation of the line along with the value of m, In this case, I will be using (3, 5). We have

`\begin{gathered} 5=((4)/(3))3+b \\ 5=4+b \\ b=5-4 \\ b=1 \end{gathered}`

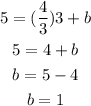
We now have the values for m and b. Just put it on the slope-intercept line form to represent the equation of the line

`y=(4)/(3)x+1`

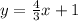
Answer: y = (4/3)x + 1