Question

Consider the equation log (3x-1)=log base 2 of 8. Describe the steps you would take to solve the equation, and state what 3x-1 is equal to.

Answer 1

The bases are not the same, so you cannot set 3x - 1 equal to 8.

You can evaluate the logarithm on the right side of the equation to get 3.

You can use the definition of a logarithm to write 3x - 1 = 1000.

Answer 2

Answer: The solution of the given equation is
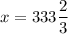`x=333(2)/(3)` and the value of (3x - 1) is 1000.

Step-by-step explanation: We are given to describe the steps in solving the following logarithmic equation:

`\log(3x-1)=\log_28.`

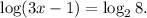
Also, we are to find the value of
`(3x-1).`

We will be using the following logarithmic properties:

`(i)~\log_ba=x~~~\Rightarrow a=b^x,\\\\(ii)~\log_ba=(\log a)/(\log b),\\\\(iii)~\log a^b=b\log a.`

We note here that if the base of logarithm is not mentioned, then we assume it to be 10.

The solution is as follows:

`\log(3x-1)=\log_28\\\\\Rightarrow \log(3x-1)=(\log8)/(\log2)\\\\\Rightarrow log(3x-1)=(\log2^3)/(\log2)\\\\\Rightarrow \log(3x-1)=(3\log2)/(\log2)\\\\\Rightarrow \log(3x-1)=3\\\\\Rightarrow 3x-1=10^3\\\\\Rightarrow 3x-1=1000\\\\\Rightarrow 3x=1001\\\\\Rightarrow x=(1001)/(3)\\\\\Rightarrow x=333(2)/(3).`

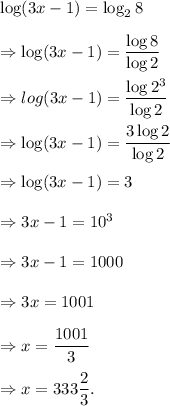
Thus, the solution of the given equation is
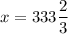`x=333(2)/(3)` and the value of (3x - 1) is 1000.