Answer: The correct option is graph (A).
Step-by-step explanation: We are given to select the graph that represents the following linear equation :

Comparing with the slope-intercept form y = mx + c of a straight line, we see that
the slope of the line (i) is
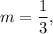 and y-intercept, c = -4.
and y-intercept, c = -4.
Also, we know that the slope of a line passing through the points (a, b) and (c, d) is given by

Graph (A) :
The points (0, -4) and (3, -3) lies on the line. So, its slope will be
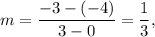
the point where the line crosses the y-axis is (0, -4).
That is, y-intercept, c = -4.
Since the slope and y-intercept of graph (A) is same as that of line (i), so this option is CORRECT.
Graph (B) :
The points (0, -2) and (3, -3) lies on the line. So, its slope will be
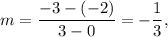
Since the slope of graph (B) is not same as that of line (i), so this option is NOT CORRECT.
Graph (C) :
The points (0, -4) and (-3, 3) lies on the line. So, its slope will be
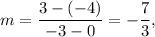
Since the slope of graph (C) is not same as that of line (i), so this option is NOT CORRECT.
Graph (D) :
The points (-4, 0) and (-3, 3) lies on the line. So, its slope will be

Since the slope of graph (B) is not same as that of line (i), so this option is NOT CORRECT.
Thus, graph (A) is the correct option.