Question

Solve the following equation by completing the square:

x^4 – 4x^2 + 2 = 0

Please show steps, and if you can, leave the numbers as fractions and sqrts instead of decimals. Thanks

Answer 1

Let
`u= x^(2)`
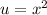
so our equation becomes
`u^(2)-4u+2=0`
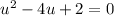
subtract 2 from both sides of the equation
`u^(2)-4u = -2`
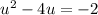
Take half of b, square the result and add to both sides
`(1)/(2)(-4) = -2` (remember -2 it will be used when factoring the trinomial)
(-2)² = 4

`u^(2)-4u+4=-2+4`
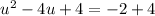
Factor trinomial and simplify right side
`(u-2)^(2)=2` note:-2 is in the factor
Square root both sides (must use +/-):
`u-2=+/- √(2)`
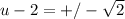
Add 2 to both sides:
`u = 2 +/- √(2)`
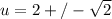
:-( not finish yet

`u= x^(2)`

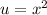
`2+ √(2) = x^(2)` and
`2- √(2) = x^(2)`
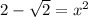
Final answers:

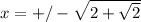
`x= +/-\sqrt{2+ √(2)}` and
`x = +/-\sqrt{2- √(2) }`

`\sqrt{2+√(2)},-\sqrt{2+√(2)},\sqrt{2-√(2)},-\sqrt{2-√(2)}`

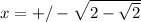
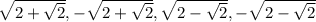
Think of it as four possible answers: