Question

Calculate the speed of the water jet emerging from the nozzle

Answer 1

Given:

• Height of ladder = 9.7 m

,

• Diameter of water hose = 2.7 inch

,

• Diameter of pump outlet = 3.47 inch

,

• Guage of water pump = 236.49 kPa = 236.49 x 10³ Pa

,

• Density of water = 1000 kg/m³

Let's find the speed of the water jet emerging from the nozzle.

To find the speed of the water jet, apply Bernoulli's equation.

We have:

`(1)/(2)p(v^2_N-v^2_p)=p_(pumpguage)-pgh`

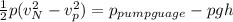
Rewrite the formula for VN:

`\begin{gathered} v^2_N-v^2_p=((2)/(p))p_(pumpguage)-2gh_{}_{} \\ \\ v^2_N-((A_N)/(A_P))^2v^2_p=((2)/(p))p_(pumpguage)-2gh \\ \\ v^2_N-(\frac{r^4_N}{r^4P_{}})^{}v^2_p=((2)/(p))p_(pumpguage)-2gh \\ \\ \\ v_N=\sqrt[]{(((2)/(p))p_(pumpguage)-2gh)/(1-((r^4_N)/(r^4_p)))} \\ \end{gathered}`

• VN is the speed of the water jet from nozzle.

,

• g is acceleration due to gravity = 9.8 m/s²

,

• h is the height of ladder = 9.7 m

,

• rN is the radius of nozzle = diameter of noozle/2 = 2.7/2 = 1.35 inches

,

• rp is the radius of the pump outlet = diameter of pump outlet/2 = 3.47/2 = 1.735 inches

,

• p is density of water = 1000 kg/m³

Input values into the formula and solve for vN:

`\begin{gathered} v_N=\sqrt[]{(((2)/(1000))(236.49*10^3)-2(9.8)(9.7))/(1-((1.35)/(1.735))^4)} \\ \\ v_N=21.1315\text{ m/s} \end{gathered}`

Therefore, the speed of the water jet from the noozle is 21.1315 m/s

ANSWER:

21.1315 m/s