Question

Which is a stretch of an exponential decay function? f(x) = 4/5(5/4)^x f(x) =4/5(4/5)^x f(x) =5/4(4/5)^x f(x) =5/4(5/4)^x

Answer 1

Answer:choice three

Explanation:

Answer 2

The correct option is 3. The function
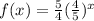`f(x)=(5)/(4)((4)/(5))^x` is a stretch of an exponential decay function.

Explanation:

The general form of an exponential function is

`f(x)=ab^x`

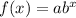
where, a is the initial value and b is the growth factor.

If b<1, then it represents a decay function and if b>1, then it represents a growth function.

Let k be a stretch and compression factor.

`g(x)=kf(x)`

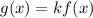
If k<1, then it represents vertical compression and if k>1, then it represents vertical stretch.

In third function

`f(x)=(5)/(4)((4)/(5))^x`

`k>1`

`b<1`

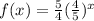
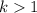
Therefore the function
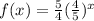`f(x)=(5)/(4)((4)/(5))^x` is a stretch of an exponential decay function.