Question

How to find the 23rd term of -21.-27.-33.-39

Answer 1

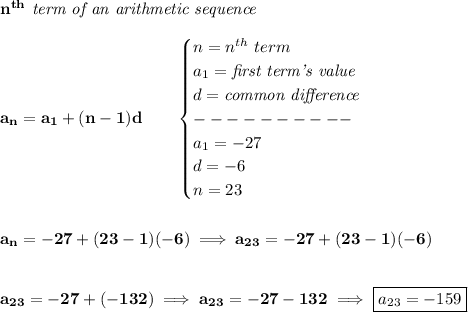
well...if you notice, the first value is -21, the second is -27... what happened? it went "down" by 6 units...ok... the next one is -33, wait a second? it went down again by 6 units? -27 - 6 = -33, the next is -39, again 6 units, what the dickens?

well, you get the next term by simply subtracting 6 or "adding" -6 to the current one, thus is an arithmetic sequence, so thus -6 is then the "common difference", and the first value is -27.


`\bf n^(th)\textit{ term of an arithmetic sequence}\\\\ a_n=a_1+(n-1)d\qquad \begin{cases} n=n^(th)\ term\\ a_1=\textit{first term's value}\\ d=\textit{common difference}\\ ----------\\ a_1=-27\\ d=-6\\ n=23 \end{cases} \\\\\\ a_n=-27+(23-1)(-6)\implies a_(23)=-27+(23-1)(-6) \\\\\\ a_(23)=-27+(-132)\implies a_(23)=-27-132\implies \boxed{a_(23)=-159}`