Answer:
The required function -
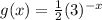
Explanation:
Given : Function represents g(x), a reflection of
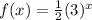 across the y-axis
across the y-axis
To find : Which function represents the g(x)?
Solution :
The function
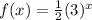
The function f(x) reflected across y-axis to form g(x)
The reflection of the point (x,y) across the y-axis is the point (-x,y).
f(x,y)→f(-x,y) , the graph of f(x) is reflected across y-axis.
Let
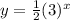
After reflection across y-axis is
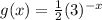
The required function.
Refer the attached graph below.