Question

How do you solve 9| x+8 | + 10 < 55 & how is it graphed

Answer 1

Hello!

First, let's write the problem.

`9\left|x+8\right|+10\ \textless \ 55|x+8|+10\ \textless \ 55`
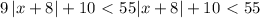
Subtract 10 from both sides.

`9\left|x+8\right|+10-10\ \textless \ 55-10`

`9\left|x+8\right|\ \textless \ 45`
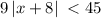
Divide both sides by 9.

`(9\left|x+8\right|)/(9)\ \textless \ (45)/(9)`

`\left|x+8\right|\ \textless \ 5`
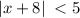
When we got absolute value, we are going to get two results.

`x+8\ \textless \ 5`
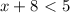
and

`x+8\ \textgreater \ -5`

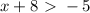
Let's solve the first one,

`x+8\ \textless \ 5x+8\ \textless \ 5`
Subtract 8 from both sides.

`x+8-8\ \textless \ 5-8`

`x\ \textless \ -3x\ \textless \ -3`

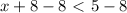
Let's solve the second one.

`x+8\ \textgreater \ -5`
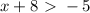
Subtract 8 from both sides.

`x+8-8\ \textgreater \ -5-8`

`x\ \textgreater \ -13x\ \textgreater \ -13`

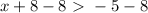
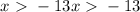
Let's combine the ranges.

`-13\ \textless \ x\ \textless \ -3`

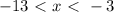
The attachment shows how would you graph it.
You can feel free to let me know if you have any questions regarding this.
Thanks!

- TetraFish