Question

A museum charges $14.50 for a one-day youth admission and $18.50 for a one-day adult admission. One Friday, the museum collected $1723 from a total of 110 youths and adults. Howmany admissions of each type were sold?The museum sold ( ) youth admissions and ( ) adult admissions.

Answer 1

Hello

To solve this question, we have to write out equations.

Let x represent youth and y represent adults

`\begin{gathered} x+y=110\ldots\text{equ(i)} \\ 14.50x+18.50y=1723\ldots\text{equ(i}i) \end{gathered}`

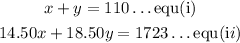
From equation (i), let's make x the subject of formula

`\begin{gathered} x+y=110 \\ x=110-y\ldots\text{equ(}iii) \end{gathered}`

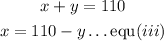
put equation (iii) into equation (ii)

`\begin{gathered} x=110-y \\ 14.50x+18.50y=1723_{} \\ 14.50(110-y)+18.50y=1723 \\ 1595-14.50y+18.50y=1723 \\ \text{collect like terms} \\ 18.50y-14.50y=1723-1595 \\ 4y=128 \\ \text{divide both sides by the coefficient of y} \\ (4y)/(4)=(128)/(4) \\ y=32 \end{gathered}`

now we havethe value of y, let's input this in equation (i) to solve for x

`\begin{gathered} x+y=110 \\ y=32 \\ x+32=110 \\ \text{collect like terms} \\ x=110-32 \\ x=78 \end{gathered}`

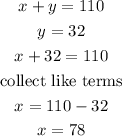
From the calculations above, the tickets sold for youth is 78 and adult is 32