Question

A professional baseball diamond is a square. The distance from base to base is 90 ft. To the nearest foot, how far does a catcher standing at home plate throw the ball across the diagonal of the square to second base?

Group of answer choices

64 feet

127 feet

94 feet

180 feet

Answer 1

Let's assume the question as per the conception of diagram provided in attachment.

We have given that we are having a baseball diamond which is a square. Let that given square be ABCD. Distance from base to base means that the length of side of square is 90ft. As all sides of square are equal then measure of all sides of square are 90ft and we have asked to find out the length of the diagonal (BD) through which ball should cross. We have given data provided is that,

• Length of sides (s) = 90Ft

• Diagonal (BD) = ?

We know the standard formula which is,

`\implies\rm{s = (d)/( √(2) ) }`

Substituting values in given formula,

`\implies\rm{90 = (d)/( √(2) ) }`

`\implies\rm{d = 90 * √(2) }`

`\implies\rm{d = 90 √(2) }`

`\implies\rm{d = 90 * 1.42 \: ... \: ( √(2) = 1.42)}`

`\implies\rm{d = 127.8}`

`\implies\rm{d = 127}`

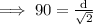
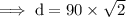
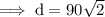
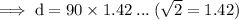
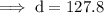
• Do the correct answer is option B) which is 127ft

Answer 2

BSE is square.

Let side be s

• s=90ft

Diagonal be d

`\\ \tt\longmapsto s=(d)/(√(2))`

`\\ \tt\longmapsto d=s√(2)`

`\\ \tt\longmapsto d=90√(2)`

`\\ \tt\longmapsto d=127ft`