Question

Assume that the salaries of elementary school teachers in the united states are normally distributed with a mean of $32,000 and a standard deviation of $3000. if 100 teachers are randomly selected, find the probability that their mean salary is less than $32,500.

Answer 1

Can you make this question clearer to read

Answer 2

Answer: 0.9525

Explanation:

Given : The salaries of elementary school teachers in the united states are normally distributed with a mean of
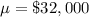`\mu=\$32,000` and a standard deviation of
`\sigma=\$3000`.

Sample size : n= 100

Let x be a random variable that denotes the salaries of elementary school teachers in the united states.

Formula :
`z=(x-\mu)/((\sigma)/(√(n)))`

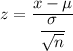
Then, the probability that their mean salary is less than $32,500 will be :-

`P(x<32500)=P((x-\mu)/((\sigma)/(√(n)))<(32500-32000)/((3000)/(√(81))))\\\\=P(z<(500)/((3000)/(10)))\approx P(z<1.67)=0.9525\ \ [\text{Using z-value}]`

Hence, the probability that their mean salary is less than $32,500 = 0.9525