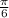Question

Triangle ABC has side lengths: √6, √2, and 2√2 units. The measures of the angles of the triangle would be... If the base of the triangle has a length of √6 then the measure of the base angle is...

Answer 1

The three sides given shows the it is a 30-60-90 right triangle. Since the base of sqrt (6) is the second largest number it would correspond to 60°.

Answer 2

the measure of the angles are:
`{(\pi)/(3),(\pi)/(6),(\pi)/(2)}`

Explanation:

Let the side of the triangle ABC is given by a,b,c.

The measure of the angle is given by as depicted in the figure.

so let
`a=√(6),b=√(2),c=2√(2)`

`A=\arccos((1)/(2)), B=\arccos((√(3))/(2)) , C=\arccos (0)`

`A=(\pi)/(3),B=(\pi)/(6),C=(\pi)/(2)`

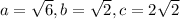
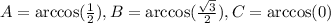
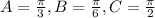
if the base of a triangle is
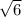`√(6)`, then let the base angle be some X.

`\cos (X)=(√(6))/(2√(2))`

where
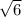`√(6)` is base and
`2√(2)` is hypotneuse.

`X=\arccos((√(3))/(2))`

`X=(\pi)/(6)`

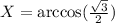
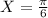
so the measure of base angle when base length is
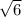`√(6)` is
`(\pi)/(6)`