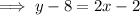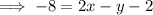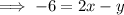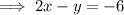Question

Find an equation of the line.Write the equation in the standard form.

Through (1,8); parallel to 2x-y=7.

Answer 1

`2x-y=-6`

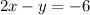
Explanation:

`\boxed{\begin{minipage}{6.3 cm}\underline{Slope-intercept form of a linear equation}\\\\$y=mx+b$\\\\where:\\ \phantom{ww}$\bullet$ $m$ is the slope. \\ \phantom{ww}$\bullet$ $b$ is the $y$-intercept.\\\end{minipage}}`

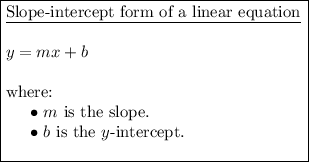
Rewrite the given equation in slope-intercept form by isolating y:

`\implies 2x-y=7`

`\implies 2x-y+y=7+y`

`\implies 2x=7+y`

`\implies 2x-7=7+y-7`

`\implies y=2x-7`

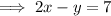
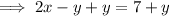
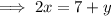
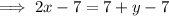
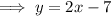
Therefore, the slope of the given equation is 2.

If two lines are parallel, their slopes are the same.

Therefore, the slope of the line parallel to the given line is 2.

`\boxed{\begin{minipage}{5.8 cm}\underline{Point-slope form of a linear equation}\\\\$y-y_1=m(x-x_1)$\\\\where:\\ \phantom{ww}$\bullet$ $m$ is the slope. \\ \phantom{ww}$\bullet$ $(x_1,y_1)$ is a point on the line.\\\end{minipage}}`

Substitute the found slope 2 and the given point (1, 8) into the point-slope formula:

`\implies y-8=2(x-1)`

`\boxed{\begin{minipage}{5.5 cm}\underline{Standard form of a linear equation}\\\\$Ax+By=C$\\\\where:\\ \phantom{ww}$\bullet$ $A, B$ and $C$ are constants. \\ \phantom{ww}$\bullet$ $A$ must be positive.\\\end{minipage}}`

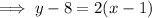
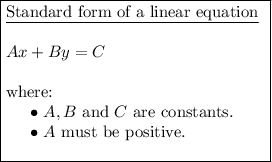
Expand the found equation and rewrite it in standard form:

`\implies y-8=2x-2`

`\implies -8=2x-y-2`

`\implies -6=2x-y`

`\implies 2x-y=-6`