Question

Please help. Consider the leading term of the polynomial function. What is the end behavior of the graph? Describe the end behavior and provide the leading term.

-3x5 + 9x4 + 5x3 + 3

Answer 1

The end behavior of graph is:

when x → -∞ f(x) → ∞

and when x → ∞ f(x) → -∞

Explanation:

We are given a polynomial function by:

`f(x)=-3x^5+9x^4+5x^3+3`

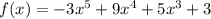
The leading term of the polynomial function is: -3 which is negative.

Also, the degree of the polynomial is odd.

• We know if the leading term of the polynomial is negative and degree of polynomial is odd then :

when x → -∞ f(x) → ∞

and when x → ∞ f(x) → -∞

• But when the leading coefficient is positive and degree of polynomial is odd then,

when x → -∞ f(x) → -∞

and when x → ∞ f(x) → ∞

• We know if the leading term of the polynomial is negative and degree of polynomial is even then :

when x → -∞ f(x) → -∞

and when x → ∞ f(x) → -∞

• But when the leading coefficient is positive and degree of polynomial is even then,

when x → -∞ f(x) → ∞

and when x → ∞ f(x) → ∞

Also, we may see the end behavior with the help of graph of the polynomial function.

Answer 2

The number with the highest exponent is the leading term, so in this case -3x^5 is the leading term.

Because the exponent is an odd number (5) the graph will rise on one side and fall on the other side.

The side that rises or falls is determined by the first number, since it is a negative number ( -3) this graph would rise on the left side and fall on the right side.