Question

Suppose sinA = 12/13 with 90º≤A≤180º. Suppose also that sinB = -7/25 with -90º≤B≤0º. Find cos(A + B).

Answer 1

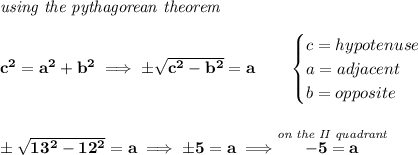
bear in mind that, the angle A is in the range of the II quadrant, where "y" is positive and "x" is negative.


`\bf sin(\theta)=\cfrac{opposite}{hypotenuse} \qquad\qquad cos(\theta)=\cfrac{adjacent}{hypotenuse} \\\\\\ \textit{also recall that }\qquad cos({{ \alpha}} + {{ \beta}})= cos({{ \alpha}})cos({{ \beta}})- sin({{ \alpha}})sin({{ \beta}})\\\\ -------------------------------\\\\ \stackrel{\textit{II quadrant}}{sin(A)}=\cfrac{\stackrel{opposite}{12}}{\stackrel{hypotenuse}{13}}\impliedby \textit{now let's find the \underline{adjacent} side}`


`\bf \textit{using the pythagorean theorem}\\\\ c^2=a^2+b^2\implies \pm√(c^2-b^2)=a\qquad \begin{cases} c=hypotenuse\\ a=adjacent\\ b=opposite\\ \end{cases} \\\\\\ \pm√(13^2-12^2)=a\implies \pm 5=a\implies \stackrel{\textit{on the II quadrant}}{-5=a}`

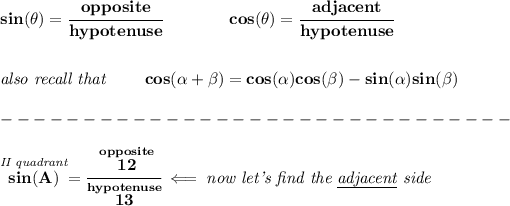
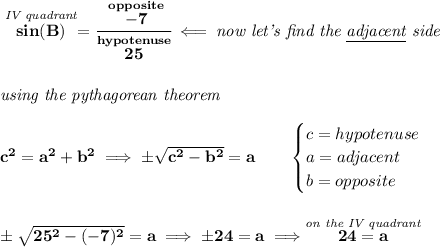
now for angle B, bear in mind it's on the IV quadrant, and "y" is negative there whilst "x" is positive.

another thing, the sine is negative, but sine is opp/hyp, now, the hypotenuse is just a radius unit, and therefore never negative, so the negative in the fraction must be the numerator, thus is -7.


`\bf \stackrel{\textit{IV quadrant}}{sin(B)}=\cfrac{\stackrel{opposite}{-7}}{\stackrel{hypotenuse}{25}}\impliedby \textit{now let's find the \underline{adjacent} side}\\\\\\ \textit{using the pythagorean theorem}\\\\ c^2=a^2+b^2\implies \pm√(c^2-b^2)=a\qquad \begin{cases} c=hypotenuse\\ a=adjacent\\ b=opposite\\ \end{cases} \\\\\\ \pm√(25^2-(-7)^2)=a\implies \pm 24=a\implies \stackrel{\textit{on the IV quadrant}}{24=a}`

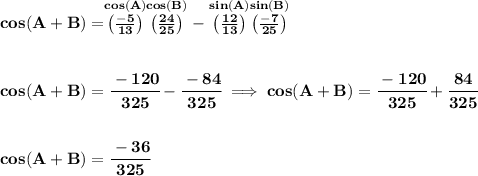
so, now, we have all sides, so lets' add cos(A + B) then


`\bf cos(A+B)=\stackrel{cos(A)}{\left( (-5)/(13) \right)}\stackrel{cos(B)}{\left( (24)/(25) \right)}-\stackrel{sin(A)}{\left( (12)/(13) \right)}\stackrel{sin(B)}{\left( (-7)/(25) \right)} \\\\\\ cos(A+B)=\cfrac{-120}{325}-\cfrac{-84}{325}\implies cos(A+B)=\cfrac{-120}{325}+\cfrac{84}{325} \\\\\\ cos(A+B)=\cfrac{-36}{325}`