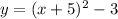we have
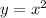
This is a vertical parabola open upward
the vertex is equal to the origin

The rule of the translation is

that means
the translations is
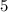 units to the left and
units to the left and
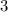 units down
units down
therefore
the new vertex of the function will be

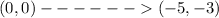
The new equation of the parabola in vertex form is equal to
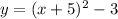
the answer is the option B