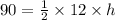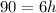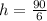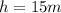Question

A triangle with an area of 10 square meters has a base of 4 meters. A similar triangle has an area of 90 square meters. What is the height of the larger triangle?

A triangle with an area of 10 square meters has a base of 4 meters. A similar triangle has an area of 90 square meters. What is the height of the larger triangle?

Answer 1

ANSWER

The height is 15 meters.

Area of smaller triangle is


`= 10 {m}^(2)`



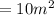
Area of larger triangle,


`= 90 {m}^(2)`


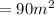
Scale factor for the area is


`{k}^(2) = (90)/(10) = 9`

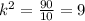
This implies that,


`k = √(9) = 3`


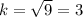
The length of the base of smaller triangle is


`= 4`

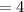
This means that the length of the base of the larger triangle is



`= 3 * 4 = 12`

Area of the larger triangle is,


`A= (1)/(2) * b * h`



`90= (1)/(2) * 12 * h`


`90= 6h`


`h = (90)/(6)`


`h = 15m`