We are given two monomials
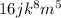
and

To find the lowest common multiple
we can express each of the monomials as follow
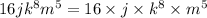

For the number part of the monomials,
The multiples of 16 are: 16, 32, 48, 64, 80, 96,-----
The multiples of 20 are : 20, 40, 60, 80, 100,-----
The lowest common multiple of 16 and 20 is 80
For the alphabet parts, the alphabet with the highest power
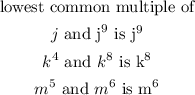
If we combine the terms to give the lowest common multiple, we will obtain

Thus, Option C is correct