Question

The following set of four ordered pairs below defines the vertices, in counterclockwise order, of a quadrilateral (four-sided figure)Find the slope of the indicated sides of the quadrilateral

Answer 1

Given the question in the image, the following are the solution steps to answer the question.

STEP 1: Write the first two vertices given

`(-5,-1),(-6,3)`

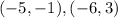
STEP 2: Find the slope

The side connecting the two given points will be gotten using distance formula;

`\begin{gathered} \mathrm{Slope\:between\:two\:points}:\quad \mathrm{Slope}=(y_2-y_1)/(x_2-x_1) \\ \left(x_1,\:y_1\right)=\left(-5,\:-1\right),\:\left(x_2,\:y_2\right)=\left(-6,\:3\right) \\ m=(3-\left(-1\right))/(-6-\left(-5\right)) \\ m=-4 \end{gathered}`

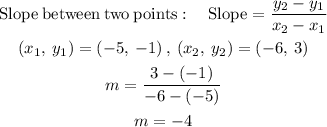
Slope is -4

STEP 3: Write the second two vertices given

`(0,1),(-1,5)`

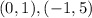
STEP 4: find the slope

`\begin{gathered} \mathrm{Slope\:between\:two\:points}:\quad \mathrm{Slope}=(y_2-y_1)/(x_2-x_1) \\ \left(x_1,\:y_1\right)=\left(0,\:1\right),\:\left(x_2,\:y_2\right)=\left(-1,\:5\right) \\ m=(5-1)/(-1-0) \\ m=-4 \end{gathered}`

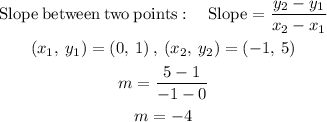
Slope is -4