1) Gathering the data
The half-life of Radium 223: 11.4 days
Initial Mass of Rd-223 : 120g
The final mass of Rd-223: 100
2) Let's use one of our possible formulas for exponential decay to calculate how long until Radium-223 gets from 120g to 100grams. And applying properties of the Logarithm
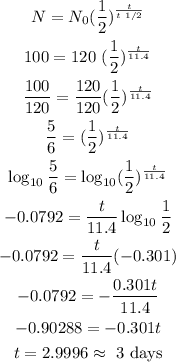
3) So it will take approximately 3 days to the Radium-223 isotope reduce to 100 grams.