Let the prime number which does not repeat be
a, the prime number which repeats twice be
b, the prime number which repeats 3 times be
c.
Then the prime factorization of this number is

.
Let's find the prime factorization of 1000:

So let c= 5, b be a number such that b^2>2^3=8, for example let b = 3, and let a be any prime, for example 2
thus the number is
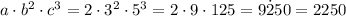
Answer: 2250