Assuming the problem as stated is, given
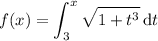
find
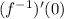
.
Provided that
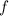
is (at least locally) invertible, we have
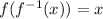
, and differentiating both sides with respect to

gives
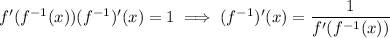
Notice that
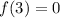
, which means
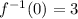
. From this it follows that
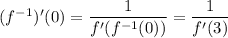
and since
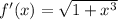
by the fundamental theorem of calculus, it follows that

, and so
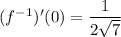
.