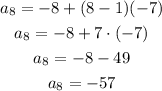Question

Finding a specify term of an arithmetic sequence given two terms

Answer 1

-57

Explanation

Arithmetic sequence formula

`a_n=a_1+(n-1)d`

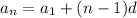
where

• aₙ is the ,nth, term

,

• a₁ is the first term

,

• n indicates the term position

,

• d is the common difference

Substituting a₁ = -8, n = 32, and the 32nd term = -225, and solving for d:

`\begin{gathered} a_(32)=a_1+(32-1)d \\ -225=-8+31\cdot d \\ -225+8=31d \\ -(217)/(31)=d \\ d=-7 \end{gathered}`

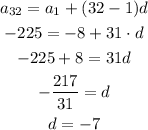
Substituting a₁ = -8, n = 8, d = -7, the value of the 8th term is:

`\begin{gathered} a_8=-8+(8-1)(-7) \\ a_8=-8+7\cdot(-7) \\ a_8=-8-49 \\ a_8=-57 \end{gathered}`