Question

A rectangular room is 9 feet longer than it is wide. The area of the room is 360 square feet. How many feet long is the room?

Answer 1

we make a drawing

where w is width

area of a rectangle is

`A=l* w`

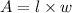
where a is tha area, l the length and w the width

then replacing the area and values of length and width

`\begin{gathered} 360=(w+9)*(w) \\ 360=w^2+9w \end{gathered}`

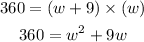
we rewrite the expression equaling 0 to find w

`\begin{gathered} w^2+9w=360 \\ w^2+9w-360=0 \end{gathered}`

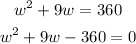
factor by quadratic formula

`w=\frac{-b\pm\sqrt[]{b^2-4ac}}{2a}`

where a is 1, b is 9 and c -360

`\begin{gathered} w=\frac{-(9)\pm\sqrt[]{(9)^2-4(1)(-360)}}{2(1)} \\ \\ w=\frac{-9\pm\sqrt[]{81+1440}}{2} \\ \\ w=\frac{-9\pm\sqrt[]{1521}}{2} \\ \\ w=(-9\pm39)/(2) \end{gathered}`

we have two solutions to w

`\begin{gathered} w_1=(-9+39)/(2)=15 \\ \\ w_2=(-9-39)/(2)=-24 \end{gathered}`

but we use the possitive number because is a measure and the measure are not negatives

the long of the room is

`w+9`

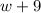
replacing w

`\begin{gathered} 15+9 \\ =24 \end{gathered}`

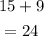
tha