Question

The second side of a triangular deck is 6 feet longer than the shortest side and a third side that is 6 feet shorter than twice the length of the shortest side. If the perimeter of the deck is 68 ​feet, what are the lengths of the three​ sides?

Answer 1

you have x, x + 6, and 2x - 6. Together, they equal 68 feet, meaning that 4x = 68. therefore x = 17 feet. The  lengths would then be 17 ft, 23 ft, and 28 ft.

Answer 2

Answer: The required lengths of the three sides of the deck are 17 feet, 23 feet and 28 feet.

Step-by-step explanation: Given that the second side of a triangular deck is 6 feet longer than the shortest side and a third side that is 6 feet shorter than twice the length of the shortest side.

The perimeter of the deck is 68 ​feet.

We are to find the lengths of the three sides of the deck.

Let x feet be the length of the shortest side.

Then, according to the given information,

the length of the second side = (x+6) feet

and

the length of the third side = (2x-6) feet.

Since the perimeter of the deck is 68 feet, so we must have

`x+x+6+2x-6=68\\\\\Rightarrow 4x=68x=(68)/(4)\\\\\Rightarrow x=17.`

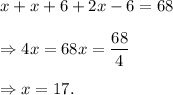
Therefore the lengths of the three sides are

17 feet, (17+6) feet and (2×17-6) feet

= 17 feet, 23 feet and 28 feet.

Thus, the required lengths of the three sides of the deck are 17 feet, 23 feet and 28 feet.