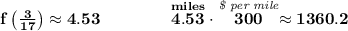Question

You are building a new house on a cartesian plane whose units are measured in miles.

Your house is to be located at the point (6,0). Unfortunately, the

existing gas line follows the curve y=√16x^(2)+6x+19.

It costs 300 dollars per mile to install new pipe connecting your house to the existing line.

What is the least amount of money you could pay to get hooked up to the system?

Answer 1

so.. we'll use the distance formula for it, keeping in mind that, where the line touches the parabola is at ( x , f(x) ), check the picture below on the left-hand-side


`\bf y=√(16x^2+6x+19)\\\\ -------------------------------\\\\ L(x)=\left[(6-x)^2+(0-√(16x^2+6x+19))^2 \right]^{(1)/(2)} \\\\\\ L(x)=[36-12x+x^2+16x^2+6x+19]^{(1)/(2)} \\\\\\ L(x)=(17x^2-6x+55)^{(1)/(2)}\\\\`

so, that's L(x) equation for the line, simplified, now, let's check its derivative.


`\bf \cfrac{dL}{dx}=\cfrac{1}{2}(17x^2-6x+55)^{-(1)/(2)}(34x-6) \\\\\\ \cfrac{dL}{dx}=\cfrac{1}{2}(17x^2-6x+55)^{-(1)/(2)}2(17x-3) \\\\\\ \cfrac{dL}{dx}=\cfrac{17x-3}{√(17x^2-6x+55)}\\\\`

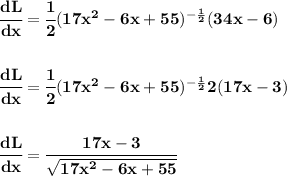
now, we get critical points when the derivative is 0, or when the denominator is 0, however (usually cusps or asymptotes), but in this case, we won't check the denominator, because it doesn't yield any, so, we'll just zero out the derivative and see what critical points we get.


`\bf 0=17x-3\implies 3=17x\implies \cfrac{3}{17}=x`

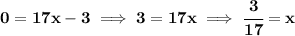
easy enough, so we only got one point... well, is it a minimum though?

well, running a first-derivative test on the left-region and the right-region of 3/17, we get a negative value on the left and a positive value on the right, that means the original function drops and then goes up, check the picture on the right-hand-side.

So, it's a minimum, those numbers in red in the picture are the ones I used to check it, but any value on the region will work, even -1,000,000, anyway.

so, at 3/17 is at a minimum... now how many miles, and how much is that?


`\bf f\left( (3)/(17) \right)\approx 4.53\qquad \qquad \stackrel{miles}{4.53}\cdot \stackrel{\textit{\$ per mile}}{300}\approx 1360.2`