Question

Find the area of the region bounded by the parabola y=2x^2 , the tangent line to the parabola at (5,50), and the x-axis

Answer 1

check the picture below.

so.. the graph looks like so, since the tangent line is at 5,50, it touches the parabola when y = 50, x = 5.

so, let's get the tangent line at that point,


`\bf y=2x^2\implies \left. \cfrac{dy}{dx}=4x \right|_(x=5)\implies 20\leftarrow m \\\\\\ y-y_1=m(x-x_1)\implies y-50=20(x-5)\implies y=20x-50\\ \left. \qquad \right. \uparrow\\ \textit{point-slope form}`

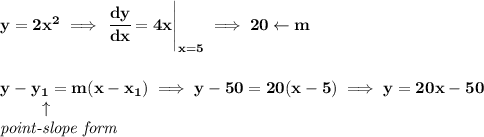
since I don't see an Up/Down function, just a Right/Left one... so, we'll use the function in "y-terms" then, the second one in the graph for each.

now... to get the bounds.... we could make them equal to each other, however, we know that the x-axis( y = 0) is the boundary line at the bottom, and that the tangent line is touching the parabolat a y = 50, thus, our bounds are from 0 to 50.


`\bf \begin{cases} \cfrac{y+50}{20}=x\impliedby \textit{right function}\\\\ \sqrt{\cfrac{y}{2}}=x\impliedby \textit{left function} \end{cases} \\\\\\ \displaystyle \int\limits_(0)^(50)~\left[ \left( \cfrac{y+50}{20} \right)~-~\left( \sqrt{\cfrac{y}{2}}\right) \right]dy \\\\\\ \displaystyle \cfrac{1}{20}\int\limits_(0)^(50)~y\cdot dy+\int\limits_(0)^(50)~\cfrac{5}{2}\cdot dy-\cfrac{1}{√(2)}\int\limits_(0)^(50)~y^{(1)/(2)}\cdot dy`


`\bf \left. \cfrac{y^2}{40}+\cfrac{5y}{2}-\cfrac{2√(y^3)}{3√(2)} \right]_(0)^(50)\implies \left[ \cfrac{250}{4}+125-\cfrac{500√(2)}{3√(2)} \right]-[0] \\\\\\ \cfrac{375}{2}-\cfrac{500}{3}\implies \cfrac{125}{6}`