Question

What is the complete factorization of the polynomial below. x^3-3x^2+x-3

Answer 1

(x-3)(x+i)(x-i)

Explanation:

`x^3-3x^2+x-3`

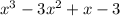
We apply grouping method

We group first two terms and last two terms

`(x^3-3x^2)+(x-3)`

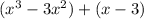
Take out GCF x^2 from first group. Also take out 1 from second group

`x^2(x-3)+1(x-3)`

`(x-3) (x^2+1)`

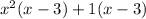
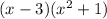
Now we factor x^2 + 1

We use difference of square formula

a^2 - b^2 = (a+b)(a-b)

x^1 + 1 can be written as
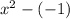`x^2 - (-1)`, the value of -1 is i^2

`x^2 +1 = x^2 -(-1) = x^2 - i^2`

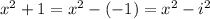
x^2 - i^2 = (x+i)(x-i)

Final answer is (x-3)(x+i)(x-i)