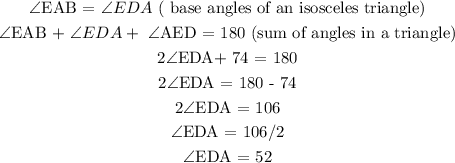Question

May I please get help with this. I have tried multiple times but still could not get the right answers

Answer 1

AE = 15

∠EDA = 52°

Step-by-step explanation:

The sahpe has been given as a rectangle.

In a rectangle, the diagonals bisect each other and they are equal to each other.

The given diagonals:

BD = 4x - 2

AC = 5x - 10

We find the value of x by equating the diagonals:

`\begin{gathered} BD\text{ = AC} \\ 4x\text{ -2 = 5x - 10} \\ 4x\text{ - 2 + 10 = 5x - 10 + 10} \\ 4x\text{ + 8 = 5x } \\ 4x\text{ -4x + 8 = 5x - 4x} \\ 8\text{ = x} \\ \\ x\text{ = 8} \end{gathered}`

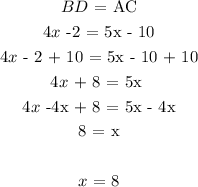
Since the diagonals bisect each other,

AE = EC

DE = EB

To find AE:

AE + EC = AC

AE + AE = AC

AC = 5x - 10 = 5(8) - 10 = 30

2AE = 30

AE = 30/2

AE = 15

To get ∠EDA:

∠EAB = 37°

`\begin{gathered} \angle EAB\text{ = }\angle EBA=37\degree\text{ (base angles of isosceles triangles are equal)} \\ Since\text{ the diagonals bisect, the triangles are isosceles triangles with two sides equal} \\ \angle\text{AEB + }\angle EAB\text{ + }\angle EBA\text{ = 180} \\ \angle\text{AEB + 37 + 37 = 180} \\ \angle\text{AEB }=\text{ 180 - 74} \\ \angle\text{AEB }=106\degree \\ \\ \angle\text{AEB + }\angle\text{AED = 180 (angles on a line)} \\ 106\text{ + }\angle\text{AED = 180} \\ \angle\text{AED = 180}-\text{ 106 = 74}\degree \end{gathered}`

`\begin{gathered} \angle\text{EAB = }\angle EDA\text{ ( base angles of an isosceles triangle)} \\ \angle\text{EAB + }\angle EDA+\text{ }\angle\text{AED = 180}\degree\text{ (sum of angles in a triangle)} \\ 2\angle\text{EDA+ 74 = 180} \\ 2\angle\text{EDA = 180 - 74} \\ 2\angle\text{EDA = 106} \\ \angle\text{EDA = 106/2} \\ \angle\text{EDA = 52}\degree \end{gathered}`