Question

F(x) = 2x + 6, g(x) = 4x2 Find (f + g)(x).

Answers are: 8x^3 + 24x
2x+6/4x^2
4x^2 + 2x + 6
-4x^2 + 2x + 6

Answer 1

hello :
F(x) = 2x + 6, g(x) = 4x²
(f + g)(x)= f(x) +g(x) = 4x²+2x+6

Answer 2

Answer: The correct option is

(C)
`4x^2+2x+6.`

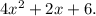
Step-by-step explanation: We are given the functions f(x) and g(x) as follows :

`f(x)=2x+6,\\\\g(x)=4x^2.`

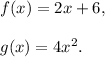
We are to find the value of (f+g)(x).

We know that

for any two functions p(x) and q(x), we have

`(p+q)(x)=p(x)+q(x).`

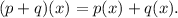
So, we get

`(f+g)(x)\\\\=f(x)+g(x)\\\\=2x+6+4x^2\\\\=4x^2+2x+6.`

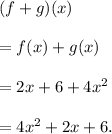
Thus, the required value is
`(4x^2+2x+6).`

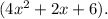
Option (C) is CORRECT.