The intermediate value theorem states that suppose f is a function continuous on every point of the interval [a, b], for any L between the values f(a) and f(b), there exist at least one number, c, in [a, b] for which f(c) = L.
83.
Given
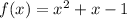
on the interval [0, 5], with f(c) = 11
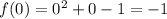
and
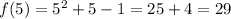
Clearly, f(c) = 11 is between f(0) = -1 and f(5) = 29
Thus, the intermediate value theorem is satisfied.
To find the value of c, we solve the equation
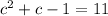
as follows:
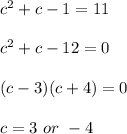
Because, c is in the interval [0, 5], therefore, the value of c is 3.
84.
Given

on the interval [0, 3], with f(c) = 0
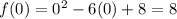
and

Clearly, f(c) = 0 is between f(0) = 8 and f(3) = -1
Thus, the intermediate value theorem is satisfied.
To find the value of c, we solve the equation
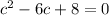
as follows:
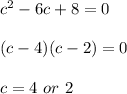
Because, c is in the interval [0, 3], therefore, the value of c is 2.
85.
Given
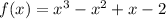
on the interval [0, 3], with f(c) = 4
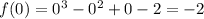
and
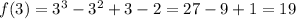
Clearly, f(c) = 4 is between f(0) = -2 and f(3) = 19
Thus, the intermediate value theorem is satisfied.
To find the value of c, we solve the equation

as follows:

Therefore, the value of c is 2.
86.
Given
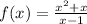
on the interval
![\left[ (5)/(2), \ 4\right]](https://img.qammunity.org/2018/formulas/mathematics/high-school/bkipsu3likqfixc47a1b5asbrsykqll9vx.png)
, with f(c) = 6
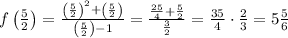
and
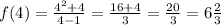
Clearly, f(c) = 6 is between
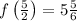
and
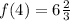
Thus, the intermediate value theorem is satisfied.
To find the value of c, we solve the equation
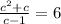
as follows:
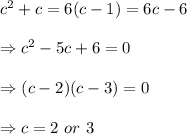
Because, c is in the interval
![\left[ (5)/(2), \ 4\right]](https://img.qammunity.org/2018/formulas/mathematics/high-school/bkipsu3likqfixc47a1b5asbrsykqll9vx.png)
, therefore, the value of c is 3.