Question

Use the following graph of the function f(x) = −3x4 − x3 + 3x2 + x + 3 to answer this question: graph of negative 3 x to the fourth, minus x cubed, plus 3 x squared, plus x plus 3 What is the average rate of change from x = 0 to x = 1?

Answer 1

The average rate of change from x=0 to x=1 for f(x) is 0.

Explanation:

We are given the function
`f(x)=-3x^(4)-x^(3)+3x^(2)+x+3`.

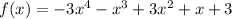Now, the rate average rate of change of a function from
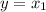`y=x_(1)` to
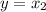`y=x_(2)` is given by
`(f(x_(2))-f(x_(1)))/(x_(2)-x_(1))`.

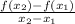As, we need the rate of change from x = 0 to x = 1.

So, we will find the values of f(0) and f(1).

i.e.
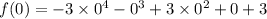`f(0)=-3*0^(4)-0^(3)+3*0^(2)+0+3` i.e. f(0) = 3

and
`f(1)=-3*1^(4)-1^(3)+3*1^(2)+1+3` i.e.
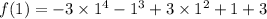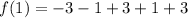`f(1)=-3-1+3+1+3` i.e. f(1) = 3

Thus, the rate of change from x=0 to x=1 is
`(f(1)-f(0))/(1-0)` i.e.
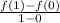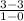`(3-3)/(1-0)` i.e. 0

Hence, the average rate of change from x=0 to x=1 for f(x) is 0.