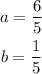Question

For what value(s) of a and b will the function below have a point discontinuities at x= -3 and x= 2

Answer 1

The value(s) of a and b for which the function f(x) have a point discontinuities at x= -3 and x= 2​ is;

`\begin{gathered} a=(6)/(5) \\ b=(1)/(5) \end{gathered}`

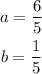
Step-by-step explanation:

The point discontinuities for the function f(x) for x= -3 is the value of a and b for which;

`2x+3=ax+3b`

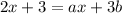
substitute x=-3, we have;

`\begin{gathered} 2(-3)+3=a(-3)+3b \\ -6+3=-3a+3b \\ -3=-3a+3b \\ \text{divide through by 3} \\ -1=-a+b \\ -a+b=-1\ldots\ldots\ldots.i \end{gathered}`

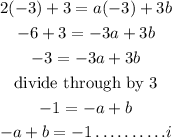
The point discontinuities for the function f(x) for x= 2 is the value of a and b for which;

`ax+3b=(-1)/(2)x^2+3x-1`

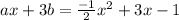
substituting x=2, we have;

`\begin{gathered} ax+3b=(-1)/(2)x^2+3x-1 \\ a(2)+3b=(-1)/(2)(2)^2+3(2)-1 \\ 2a+3b=-2+6-1 \\ 2a+3b=3\ldots\ldots\ldots\text{ }ii \end{gathered}`

So, we need to solve the simultaneous equation i and ii to get the value of a and b;

`\begin{gathered} -a+b=-1\ldots\ldots\ldots.i \\ 2a+3b=3\ldots\ldots\ldots\text{ }ii \end{gathered}`

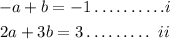
from equation i;

`b=-1+a`

substituting that into equation ii;

`\begin{gathered} 2a+3(-1+a)=3 \\ 2a-3+3a=3 \\ 5a-3=3 \\ 5a=3+3 \\ 5a=6 \\ a=(6)/(5) \end{gathered}`

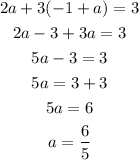
substitute the value of a to get b;

`\begin{gathered} b=-1+a \\ b=-1+((6)/(5)) \\ b=(1)/(5) \end{gathered}`

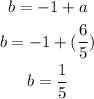
Therefore, the value(s) of a and b for which the function f(x) have a point discontinuities at x= -3 and x= 2​ is;

`\begin{gathered} a=(6)/(5) \\ b=(1)/(5) \end{gathered}`