Question

If u, v, and w are nonzero vectors in r 2 , is w a linear combination of u and v?

Answer 1

Not necessarily.
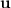
`\mathbf u` and
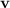
`\mathbf v` may be linearly dependent, so that their span forms a subspace of
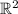
`\mathbb R^2` that does not contain every vector in
`\mathbb R^2`.

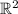
For example, we could have
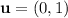
`\mathbf u=(0,1)` and
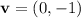
`\mathbf v=(0,-1)`. Any vector
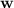
`\mathbf w` of the form
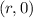
`(r,0)`, where
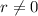
`r\\eq0`, is impossible to obtain as a linear combination of these
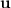
`\mathbf u` and
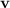
`\mathbf v`, since


`c_1\mathbf u+c_2\mathbf v=(0,c_1)+(0,-c_2)=(0,c_1-c_2)\\eq(r,0)`

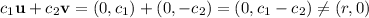
unless
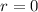
`r=0` and
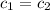
`c_1=c_2`.