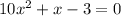Question

can you please write a quadratic equation with integer coefficients that has
`(1)/(2)`and
`- (3)/(5)`as solutions?

Answer 1

To answer this question, we need to remember that the solutions are those ones obtained when we have the quadratic equation equated to 0. The polynomial is given taking into account the inverse of the values for the solutions (or roots) as follows:

`(x-(1)/(2))\cdot(x+(3)/(5))=0`

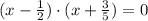
Since we need integer coefficients, we have to solve the corresponding operations inside the parentheses:

`(2x-1)\cdot(5x+3)=0`

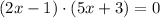
Expanding these parentheses, we have:

`(2x-1)(5x+3)=0\Rightarrow10x^2+x-3=0`

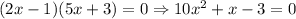
Therefore, the quadratic equation with integer coefficients is:

`10x^2+x-3=0`