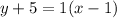Answer:
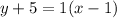
Explanation:
The point-slope form of a line is:
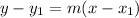 ; where
; where
 and
and
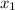 are the coordinates of a given point, and m is the slope.
are the coordinates of a given point, and m is the slope.
In addition, when two line are parallel means that they both have the same slope. So, the point-slope form we have to find it's gonna have
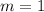 , because is parallel to a line with that slope. Also, the given point is
, because is parallel to a line with that slope. Also, the given point is
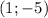 .
.
Now, we just replace all values and isolate
 :
:
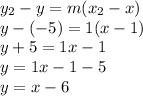
Therefore, the equation of the parallel line is:
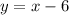
And the point-slope form is: