Question

An article in a health magazine suggested getting a dog in order to increase time spent walking (for exercise). A researcher for the article found that the distribution of time spent walking by dog-owners was approximately normally distributed with a mean of 38 minutes per day. She also found that 84% of dog-owners spend less than 45 minutes walking per day.Find the standard deviation (in minutes) for daily walking time among dog-owners.Construct a normal distribution curve that displays all relevant data for this scenario.How long would a dog-owner need to walk in a day to be ranked in the 99th percentile?

Answer 1

For normally distributed data, we can use the z-score formula:

`\begin{gathered} Z=(x-\mu)/(\sigma) \\ \text{Where } \\ z\text{ is the z-score} \\ \mu\text{ is the mean} \\ \sigma\text{ is the standard deviation} \end{gathered}`

The given information is that the mean is 38 min/day, also 84% of dog owners spend less than 45 min/day walking. This means that P(x<45)=0.84.

By checking in a standard normal table, we found that a probability of 0.84 is given by a z-score = 0.9946

By replacing this value into the z-score formula, we can solve for standard deviation as follows:

`\begin{gathered} 0.9946=(45-38)/(\sigma) \\ \sigma=(45-38)/(0.9946) \\ \sigma=(7)/(0.9946) \\ \sigma=7.03801\approx7.04 \end{gathered}`

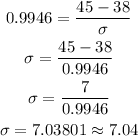
To construct the normal distribution curve, you can use the mean and the standard deviation:

In the bottom, replace the mean symbol with its value 38, and standard deviation by 7.04, and you'll get your curve.

Now, to find how long would a dog-owner need to walk in a day to be ranked in the 99th percentile, Start by finding the z-score for P(ZLooking in a standard normal table we found that z-score for 0.99 is 2.33.

Now, replace this value into the z-score formula and solve for x:

`\begin{gathered} 2.33=(x-38)/(7.03801) \\ x-38=2.33*7.03801 \\ x-38=16.3986 \\ x=16.3986+38 \\ x=54.3986\approx54.4 \end{gathered}`

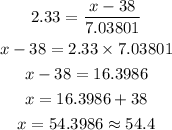
Thus, a dog-owner needs to walk 54.4 min/day to be ranked in the 99th percentile.