Question

In the figure below, ZABC = ZDEC and ZGFE - ZDCE. Point C is the point of intersectionbetween AG and BD, while point E is the point of intersection between AG and DFProve ABC - GEF

Answer 1

Given that

`\begin{gathered} \angle ABC\cong\angle DEC \\ \angle GFE\cong\angle DEC \end{gathered}`

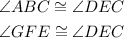
C is the point of intersection between AG and BD while E is the point of intersection between AG and DF

To prove the triangle ABC is similar to the triangle GEF

From the given figure, it is seen that

`CD=CE`

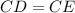
Therefore,

`\angle DEC=\angle DCE`

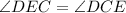
Since

`\begin{gathered} \angle ABC\cong\angle DEC \\ \angle GFE\cong\angle DEC \end{gathered}`

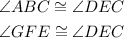
Then,

`\angle ABC\cong\angle GFE`

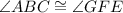
In the triangle ABC and the triangle GFE, two angles are congruent.

Therefore,

`\begin{gathered} (AC)/(BC)=(EG)/(FE) \\ (AC)/(EG)=(BC)/(FE) \end{gathered}`

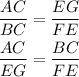
Similarly,

`(AB)/(FG)=(AC)/(EG)`

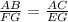
Thus,

`(AB)/(FG)=(AC)/(EG)=(BC)/(FE)`

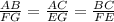
Hence, the triangle ABC is similar to the triangle GEF